Algebraic Vibrations
film
Lisans
Emeği Geçenler
- A movie by
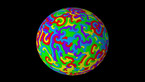
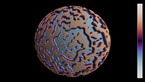
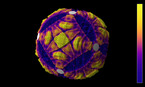
We approximate and effectively simulate different characteristic patterns of a drum vibration using algebraic surfaces.
Theoretical physicists call a drum a vibrating circular membrane. The movement of the membrane is described by Bessel functions, which are complicated, analytic functions that do not satisfy a polynomial equation.
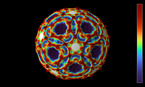
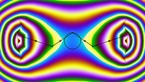
In our short movie, we approximate and effectively simulate different characteristic patterns (normal modes) of the drum vibration using algebraic surfaces instead, which are given by simple polynomial equations (in contrast to Bessel functions). The patterns are characterized by lines or circles, which stay still, while everything else is moving up and down. We show vibrations with up to three such nodal lines and two circular nodal curves.
This visualization was created with the free SURFER software by IMAGINARY. A manuscript on the math behind it is currently in preparation and will be made available on the IMAGINARY platform as well. All necessary formulas will be included to be able to create your own algebraic vibrations.