LPDJLQH D VHFUHW
film
Emeği Geçenler
- Idea
- Conception
- Realization and Production
- Surfer Movies
- Original Music
An artistic journey into the world of elliptic curve cryptography.
Pythagorean triples such as (3, 4, 5) or (4961, 6480, 8161) were well known by ancient Babylonians around 1600 B. C. They were also aware of their correspondence to right triangles with integer sides and to the problem of splitting a given square number into two squares. Although such triples have been studied in detail since the time of Euclid, around 300 B. C., it was only in the middle of the XVII century that Pierre de Fermat stated the famous observation: “No cube can be split into two cubes, nor any biquadrate into two biquadrates, nor generally any power beyond the second into two of the same kind”.
This became the famous “Fermat’s Last Theorem”, stating that the equation AN + BN = CN has no nonzero integer solutions when N is greater than 2. It was completely proven in 1994, about three and a half centuries later, using the XX century theory of elliptic curves!
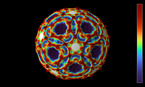
Elliptic curves have deep and beautiful properties. They are plane curves of the type y2 = x3 + a·x + b that have been studied since the XIX century. That equation in the affine plane corresponds to the homogeneous equation y2·z= x3 + a·x·z2 + b·z3, which describes in space a family of algebraic surfaces with two parameters a and b. The computational variation of these equations generates beautiful animations that stimulate our imagination and evoke our mathematical creativity.
Cryptography refers to secure methods to transmit and safeguard secret and valuable information. Since 1977 the RSA public key system has been widely used. It is based on prime number theory and on the difficulty of factoring very large integers. With the impact of the elliptic curve method for integer factorization, Elliptic Curve Cryptography (ECC) was invented by mathematicians in 1985, and since then the mathematical sophistication of cryptography has been raised to a whole new level.
The security of the ECC algorithms is based on the discrete logarithm problem of elliptic curves, which seems to be a much harder problem in finite field arithmetic. Recent mathematical advances imply that a certain desired security level can be attained with significantly smaller keys, for instance, a 160-bit ECC key provides the same level of security as a 1024-bit RSA key.
The theory of elliptic curves illustrates the beauty of the links between number theory, algebra and geometry and provides a powerful mathematical tool to strengthen security of e-commerce and secure communications. The old and unreliable method of the Caesar cipher of using only the simple arithmetic operation to encipher a message in the usual Latin alphabet by means of the formula d = c - 3 (mod 26) is outdated. But, it gives us the key to decipher the title of this film:
_ _ _ _ _ _ _ _ _ _ _ _ _ _
To download the film in high resolution in Portuguese, German, English and Spanish please go to: