Constrained Willmore surfaces in 3-space
Constrained Willmore surfaces in 3-space

Experimental constant mean curvature surface of genus 2
Different views of a constant mean curvature surface in the 3-sphere based on Lawson’s genus 2 minimal surface, analogous to a Delaunay torus. It was recently (2013) discovered by Sebastian Heller and Nicholas Schmitt using Hitchin abelianization and the Dorfmeister-Pedit-Wu (DPW) Method.

Experimental constant mean curvature surface of genus 2
Different views of a constant mean curvature surface based on Lawson’s genus 2 minimal surface, analogous to a Delaunay torus. It was recently (2013) discovered by Sebastian Heller and Nicholas Schmitt using Hitchin abelianization and the Dorfmeister-Pedit-Wu (DPW) Method.
Experimental constant mean curvature surface of genus 2
Different views of a constant mean curvature surface based on Lawson’s genus 2 minimal surface, analogous to a Delaunay torus. It was recently (2013) discovered by Sebastian Heller and Nicholas Schmitt using Hitchin abelianization and the Dorfmeister-Pedit-Wu (DPW) Method.
Experimental constant mean curvature surface of genus 2
This is a cut-through view of a constant mean curvature surface (critical surface for the area with fixed enclosed volume) based on Lawson’s genus 2 minimal surface, analogous to a Delaunay torus. It was recently (2013) discovered by Sebastian Heller and Nicholas Schmitt using Hitchin abelianization and the Dorfmeister-Pedit-Wu (DPW) Method.
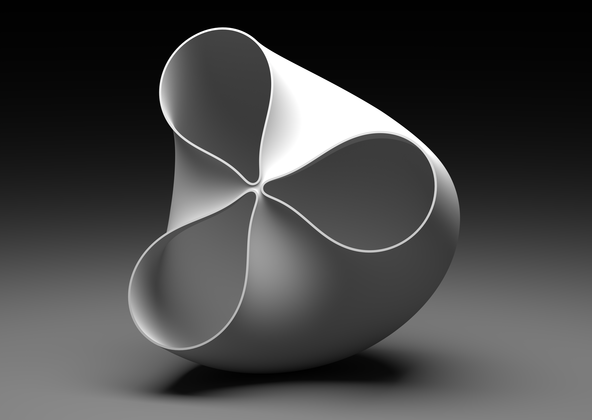
Constrained Willmore Hopf torus
This image shows constrained Willmore surfaces (critical surface for the bending energy under angle preserving deformation) in 3-space. To be precise, it is a 2-lobed constrained Willmore hopf torus of non rectangular conformal type. These are conjectured minimizers of the Willmore energy in their respective conformal type. It was discovered by Lynn Heller, Martin Kilian, Franz Pedit and Nicholas Schmitt (2012).

Lawson's genus 2 minimal surface
The Lawson surface is the only known minimal surface of genus two in the 3-sphere. It was found by Blaine Lawson Jr. in 1970. It has various symmetries and is build out of a single piece by rotation and reflection. The lines show a conformal asymptotic line parametrization of the surface found by Sebastian Heller and Nicholas Schmitt in 2013
Wente torus
The Wente torus, the first known example of an immersed torus in Euclidean space with constant mean curvature, was found by Henry Wente in 1985 via analytic methods. Earlier, Hopf had conjectured that the round sphere was the only compact and immersed constant mean curvature surface in Euclidean space. Uwe Abresch was the first to visualize this torus by computer.

Dobriner torus
A constant mean curvature torus with spectral genus 3 and rectangular conformal type. The coordinates of this torus were computed by Hermann Dobriner in his thesis in the 19th century. It is a counterexample to the Hopf conjecture. Before computer visualization, no one noticed the surface is actually compact.
Singly Periodic
Periodic surfaces of constant curvature: twizzler, breather, unduloid, nodoid
Twisted Pseudosheres
Dini surfaces in the associate family of the pseudosphere